Triple Count: 2809173894
URI Count: 391273031
Average URI length: 29.08, Standard Deviation: 13.19
Average URI reuse: 18.98
Appeared as (ignoring literals):
S only: 101791597
P only: 104
S and P: 0
O only: 39637426
O and S: 249843881
P and O: 23
S, P and O: 0
O including literals: 93843528
Literal Count: 54206102
Average literal length: 158.32, Standard Deviation: 301.96
Average literal reuse: 18.45
Blank Node Count: 0
Average Blank Node reuse: 0.00
Node appearances as S, P, O, SP, PO, OS
Aggregate node reuse
Node lengths
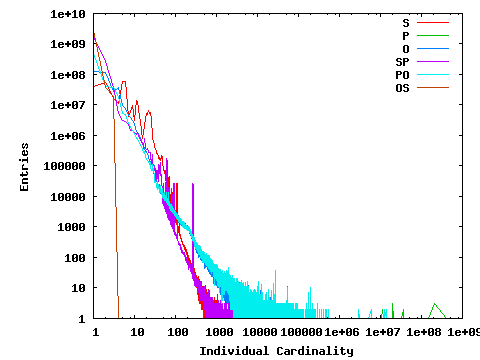
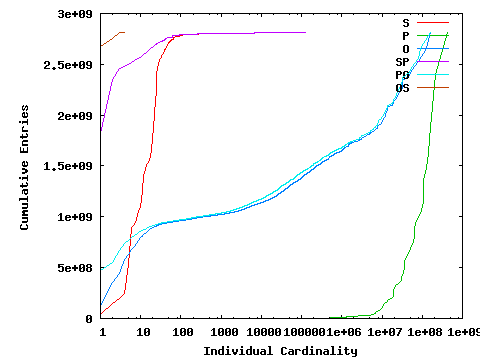
Graph 1 shows the number of times nodes (or node pairs) of a given cardinality appear. So, if there are 200,000 nodes that appear as a Subject on three occasions, then 200,000 will be plotted at an x-position of 3 on the graph.
Graph 2 is more complex: it shows the cumulative entries to give a more readable graph. In this graph, if we have 100,000 nodes that appear as a Subject only once, and 100,000 nodes that appear as a Subject twice, then we plot points at (x=1,y=100,000), and (x=2,y=300,000). Thus, if a given Subject exists many times relative to the size of the dataset, it will cause a pronounced upward tick in the graph. This second graph is useful for showing the proportion of an index over S (or P, or SP, etc) that will be made up of small entries, vs large ones with repeating elements.


| Cardinality | S | P | O | SP | PO | OS |
|---|---|---|---|---|---|---|
| Total | 351635478 | 127 | 343687432 | 2151619560 | 591661013 | 2734351818 |
| 1-1 | 39749143 | 0 | 122422328 | 1822170403 | 470540556 | 2678549398 |
| 2-2 | 55590683 | 0 | 117036507 | 263473659 | 41731214 | 36782765 |
| 3-3 | 16523708 | 0 | 27114367 | 37550409 | 38137671 | 19019654 |
| 4-4 | 11430341 | 0 | 36518018 | 5194729 | 17976442 | 1 |
| 5-5 | 57528465 | 0 | 9400196 | 3206989 | 5296608 | 0 |
| 6-6 | 60388594 | 0 | 7733445 | 2982967 | 4789474 | 0 |
| 7-7 | 4237137 | 0 | 4443999 | 2370572 | 2562360 | 0 |
| 8-8 | 6295105 | 0 | 4032765 | 1504296 | 1797058 | 0 |
| 9-9 | 9511368 | 0 | 3219638 | 1436581 | 1462460 | 0 |
| 10-19 | 46805887 | 1 | 9085318 | 7749391 | 5252901 | 0 |
| 20-29 | 37557316 | 1 | 1516494 | 2304913 | 1082105 | 0 |
| 30-39 | 3034431 | 0 | 432044 | 622738 | 286698 | 0 |
| 40-49 | 1622760 | 0 | 157085 | 537959 | 146500 | 0 |
| 50-59 | 627111 | 0 | 90547 | 112841 | 93396 | 0 |
| 60-69 | 340188 | 0 | 64624 | 216317 | 78020 | 0 |
| 70-79 | 163815 | 0 | 51958 | 29945 | 45870 | 0 |
| Cardinality | S | P | O | SP | PO | OS |
| 80-89 | 46495 | 0 | 38348 | 38188 | 34461 | 0 |
| 90-99 | 32414 | 0 | 30531 | 60174 | 30041 | 0 |
| 100-199 | 113481 | 1 | 143728 | 21460 | 141586 | 0 |
| 200-299 | 32346 | 1 | 53746 | 30725 | 55520 | 0 |
| 300-399 | 1511 | 1 | 19119 | 1273 | 20783 | 0 |
| 400-499 | 661 | 2 | 11004 | 573 | 12497 | 0 |
| 500-599 | 389 | 1 | 7632 | 366 | 9208 | 0 |
| 600-699 | 272 | 1 | 5914 | 255 | 7073 | 0 |
| 700-799 | 167 | 0 | 4419 | 156 | 5510 | 0 |
| 800-899 | 112 | 1 | 3538 | 108 | 4466 | 0 |
| 900-999 | 97 | 0 | 2815 | 97 | 3365 | 0 |
| 1000-1099 | 1 | 0 | 42 | 1 | 39 | 0 |
| 1000-1999 | 522 | 8 | 15570 | 519 | 20459 | 0 |
| 2000-2999 | 350 | 1 | 7177 | 350 | 9697 | 0 |
| 3000-3999 | 233 | 2 | 5196 | 233 | 5356 | 0 |
| 4000-4999 | 205 | 4 | 3474 | 203 | 3687 | 0 |
| 5000-5999 | 85 | 1 | 2218 | 85 | 2617 | 0 |
| Cardinality | S | P | O | SP | PO | OS |
| 6000-6999 | 30 | 0 | 1377 | 30 | 1652 | 0 |
| 7000-7999 | 19 | 1 | 1028 | 19 | 1217 | 0 |
| 8000-8999 | 6 | 1 | 841 | 6 | 948 | 0 |
| 9000-9999 | 6 | 0 | 708 | 6 | 807 | 0 |
| 10000-19999 | 16 | 5 | 3669 | 16 | 4725 | 0 |
| 20000-29999 | 1 | 2 | 1904 | 1 | 2140 | 0 |
| 30000-39999 | 2 | 3 | 995 | 2 | 912 | 0 |
| 40000-49999 | 2 | 5 | 669 | 2 | 590 | 0 |
| 50000-59999 | 0 | 1 | 448 | 0 | 343 | 0 |
| 60000-69999 | 2 | 2 | 215 | 2 | 293 | 0 |
| 70000-79999 | 0 | 1 | 184 | 0 | 241 | 0 |
| 80000-89999 | 0 | 0 | 193 | 0 | 219 | 0 |
| 90000-99999 | 0 | 1 | 139 | 0 | 118 | 0 |
| 100000-199999 | 1 | 1 | 684 | 1 | 570 | 0 |
| 200000-299999 | 0 | 8 | 188 | 0 | 178 | 0 |
| 300000-399999 | 0 | 1 | 94 | 0 | 90 | 0 |
| 400000-499999 | 0 | 2 | 61 | 0 | 58 | 0 |
| Cardinality | S | P | O | SP | PO | OS |
| 500000-599999 | 0 | 2 | 41 | 0 | 35 | 0 |
| 600000-699999 | 0 | 3 | 23 | 0 | 17 | 0 |
| 700000-799999 | 0 | 3 | 15 | 0 | 19 | 0 |
| 800000-899999 | 0 | 0 | 12 | 0 | 9 | 0 |
| 900000-999999 | 0 | 0 | 10 | 0 | 10 | 0 |
| 1000000-1999999 | 0 | 9 | 62 | 0 | 54 | 0 |
| 2000000-2999999 | 0 | 0 | 11 | 0 | 12 | 0 |
| 3000000-3999999 | 0 | 1 | 9 | 0 | 8 | 0 |
| 4000000-4999999 | 0 | 0 | 5 | 0 | 4 | 0 |
| 5000000-5999999 | 0 | 2 | 3 | 0 | 3 | 0 |
| 6000000-6999999 | 0 | 1 | 4 | 0 | 6 | 0 |
| 7000000-7999999 | 0 | 4 | 5 | 0 | 5 | 0 |
| 8000000-8999999 | 0 | 1 | 1 | 0 | 3 | 0 |
| 9000000-9999999 | 0 | 2 | 3 | 0 | 3 | 0 |
| 10000000-19999999 | 0 | 12 | 15 | 0 | 13 | 0 |
| 20000000-29999999 | 0 | 3 | 3 | 0 | 5 | 0 |
| 30000000-39999999 | 0 | 7 | 4 | 0 | 3 | 0 |
| Cardinality | S | P | O | SP | PO | OS |
| 40000000-49999999 | 0 | 1 | 1 | 0 | 1 | 0 |
| 50000000-59999999 | 0 | 1 | 1 | 0 | 1 | 0 |
| 60000000-69999999 | 0 | 4 | 0 | 0 | 0 | 0 |
| 80000000-89999999 | 0 | 0 | 1 | 0 | 1 | 0 |
| 90000000-99999999 | 0 | 1 | 0 | 0 | 1 | 0 |
| 100000000-199999999 | 0 | 6 | 2 | 0 | 1 | 0 |
| 200000000-299999999 | 0 | 3 | 0 | 0 | 0 | 0 |
| 400000000-499999999 | 0 | 1 | 0 | 0 | 0 | 0 |
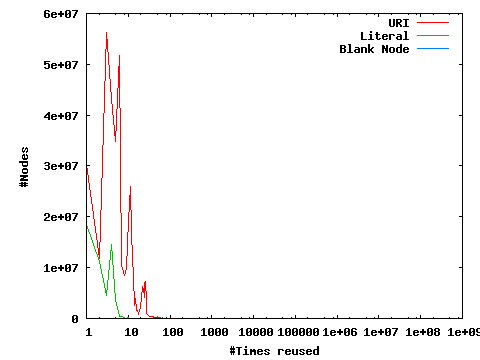
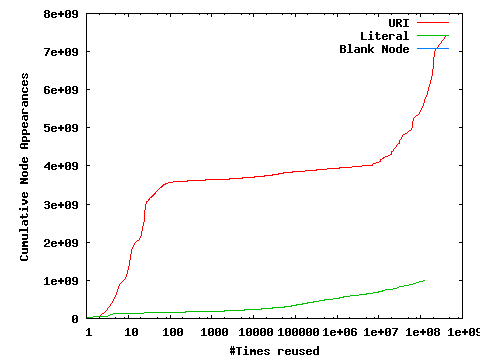
These graphs illustrate the number of times nodes are reused across all elements of a triple. Graph 1 shows the number of nodes that have been reused a given number of times: if 10 nodes appear 100 times, a point will be plotted at (x=100,y=10). Graph 2 is again more complex: if 10 nodes appear 100 times, and 2 nodes appear 101 times, points will be plotted at (x=100,y=1000), and (x=101,y=1202). This aids in visualising what proportion of the dataset is made up of heavily reused nodes vs rarely reused nodes.
Data Files: URI Literal B-Node
| #Times reused | URI | Literal | Blank Node |
|---|---|---|---|
| Total | 391273031 | 54206102 | 0 |
| 1-1 | 30238413 | 18507119 | 0 |
| 2-2 | 11838128 | 11128387 | 0 |
| 3-3 | 56231643 | 4545853 | 0 |
| 4-4 | 43321078 | 14607056 | 0 |
| 5-5 | 34746557 | 3650391 | 0 |
| 6-6 | 51788561 | 322830 | 0 |
| 7-7 | 10446355 | 296079 | 0 |
| 8-8 | 8530234 | 100995 | 0 |
| 9-9 | 9769873 | 89455 | 0 |
| 10-19 | 81829719 | 448770 | 0 |
| 20-29 | 41641375 | 151686 | 0 |
| 30-39 | 4324233 | 73589 | 0 |
| 40-49 | 2703065 | 43194 | 0 |
| 50-59 | 1649321 | 29750 | 0 |
| 60-69 | 919664 | 21900 | 0 |
| 70-79 | 568046 | 16761 | 0 |
| #Times reused | URI | Literal | Blank Node |
| 80-89 | 202983 | 13348 | 0 |
| 90-99 | 103007 | 11801 | 0 |
| 100-199 | 282185 | 69325 | 0 |
| 200-299 | 68354 | 33570 | 0 |
| 300-399 | 16432 | 8437 | 0 |
| 400-499 | 8486 | 5091 | 0 |
| 500-599 | 5708 | 3421 | 0 |
| 600-699 | 4098 | 2622 | 0 |
| 700-799 | 2975 | 1920 | 0 |
| 800-899 | 2260 | 1518 | 0 |
| 900-999 | 1768 | 1232 | 0 |
| 1000-1099 | 10 | 15 | 0 |
| 1000-1999 | 9527 | 6562 | 0 |
| 2000-2999 | 4173 | 2967 | 0 |
| 3000-3999 | 3154 | 2071 | 0 |
| 4000-4999 | 2110 | 1377 | 0 |
| 5000-5999 | 1402 | 906 | 0 |
| #Times reused | URI | Literal | Blank Node |
| 6000-6999 | 890 | 591 | 0 |
| 7000-7999 | 714 | 408 | 0 |
| 8000-8999 | 590 | 371 | 0 |
| 9000-9999 | 466 | 311 | 0 |
| 10000-19999 | 2223 | 1580 | 0 |
| 20000-29999 | 1091 | 819 | 0 |
| 30000-39999 | 720 | 283 | 0 |
| 40000-49999 | 430 | 243 | 0 |
| 50000-59999 | 236 | 210 | 0 |
| 60000-69999 | 135 | 84 | 0 |
| 70000-79999 | 77 | 109 | 0 |
| 80000-89999 | 53 | 140 | 0 |
| 90000-99999 | 47 | 94 | 0 |
| 100000-199999 | 196 | 490 | 0 |
| 200000-299999 | 69 | 128 | 0 |
| 300000-399999 | 39 | 56 | 0 |
| 400000-499999 | 20 | 41 | 0 |
| #Times reused | URI | Literal | Blank Node |
| 500000-599999 | 14 | 28 | 0 |
| 600000-699999 | 12 | 13 | 0 |
| 700000-799999 | 8 | 9 | 0 |
| 800000-899999 | 5 | 8 | 0 |
| 900000-999999 | 3 | 7 | 0 |
| 1000000-1999999 | 19 | 49 | 0 |
| 2000000-2999999 | 6 | 7 | 0 |
| 3000000-3999999 | 5 | 4 | 0 |
| 4000000-4999999 | 0 | 5 | 0 |
| 5000000-5999999 | 0 | 1 | 0 |
| 6000000-6999999 | 1 | 2 | 0 |
| 7000000-7999999 | 7 | 1 | 0 |
| 8000000-8999999 | 2 | 0 | 0 |
| 9000000-9999999 | 2 | 3 | 0 |
| 10000000-19999999 | 19 | 5 | 0 |
| 20000000-29999999 | 8 | 1 | 0 |
| 30000000-39999999 | 7 | 1 | 0 |
| #Times reused | URI | Literal | Blank Node |
| 40000000-49999999 | 1 | 1 | 0 |
| 50000000-59999999 | 2 | 0 | 0 |
| 60000000-69999999 | 4 | 0 | 0 |
| 70000000-79999999 | 1 | 0 | 0 |
| 90000000-99999999 | 1 | 0 | 0 |
| 100000000-199999999 | 7 | 1 | 0 |
| 200000000-299999999 | 3 | 0 | 0 |
| 400000000-499999999 | 1 | 0 | 0 |
These graphs illustrate the length in bytes of nodes. In both cases, even if a Node is reused many times, it is only considered once in these graphs. Graph 1 shows the number of nodes that have a given length: if 10 nodes have a length of 100 bytes, a point will be plotted at (x=100,y=10). Graph 2 is again more complex, plotting the cumulative space used: if there are 10 nodes of length 100 bytes, and 2 nodes of length 110 bytes, points will be plotted at (x=100,y=1000), and (x=110,y=1220). This aids in visualising what proportion of space is taken up by nodes of a given size.
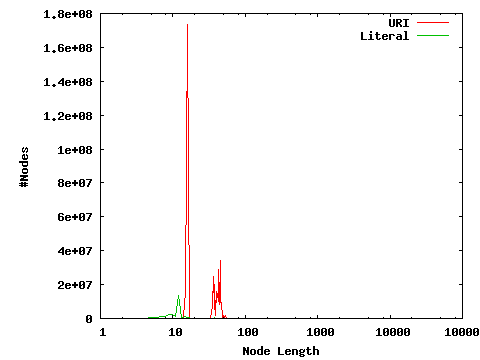
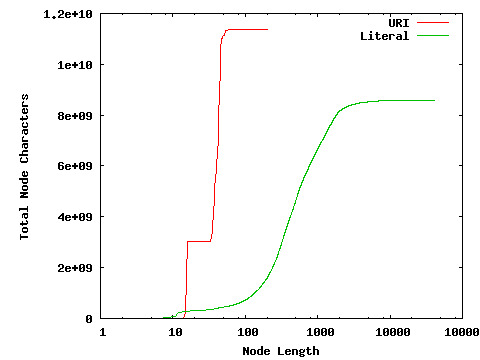
| Node Length | URI | Literal |
|---|---|---|
| Total | 391273031 | 54206101 |
| 1-1 | 0 | 64 |
| 2-2 | 0 | 2190 |
| 3-3 | 0 | 31265 |
| 4-4 | 0 | 191842 |
| 5-5 | 0 | 375910 |
| 6-6 | 0 | 596624 |
| 7-7 | 0 | 1031449 |
| 8-8 | 0 | 1193425 |
| 9-9 | 0 | 2101651 |
| 10-19 | 190439573 | 20780878 |
| 20-29 | 124 | 1672825 |
| 30-39 | 63918056 | 1560708 |
| 40-49 | 131036014 | 1207753 |
| 50-59 | 5695675 | 750303 |
| 60-69 | 161304 | 701346 |
| 70-79 | 7430 | 762338 |
| Node Length | URI | Literal |
| 80-89 | 8280 | 710313 |
| 90-99 | 2293 | 684261 |
| 100-199 | 4265 | 5954322 |
| 200-299 | 17 | 4616940 |
| 300-399 | 0 | 3200410 |
| 400-499 | 0 | 1831947 |
| 500-599 | 0 | 1203891 |
| 600-699 | 0 | 696823 |
| 700-799 | 0 | 473241 |
| 800-899 | 0 | 351584 |
| 900-999 | 0 | 278286 |
| 1000-1099 | 0 | 2449 |
| 1000-1999 | 0 | 1090341 |
| 2000-2999 | 0 | 110266 |
| 3000-3999 | 0 | 23946 |
| 4000-4999 | 0 | 8578 |
| 5000-5999 | 0 | 4627 |
| Node Length | URI | Literal |
| 6000-6999 | 0 | 1245 |
| 7000-7999 | 0 | 811 |
| 8000-8999 | 0 | 383 |
| 9000-9999 | 0 | 282 |
| 10000-19999 | 0 | 372 |
| 20000-29999 | 0 | 141 |
| 30000-39999 | 0 | 69 |
| 40000-49999 | 0 | 2 |